2014-2015 Math Highlights: Hands-On Math

My 2014-2015 Highlights list would not be complete without sharing our highlights from math this past year. It involves a lot of great hands-on math ideas, so I hope you enjoy!

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

Note: Some of the

links in this post are affiliate links. This means that I am a participant in the Amazon Services LLC Associates Program, an affiliate advertising program designed to provide a means for sites to earn advertising fees by advertising and linking to amazon.com. Read my full disclosure here.

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

Recall how my new school has a STEAM and project-based learning focus. I wanted to incorporate engineering, project-based learning, and hands-on math activities as much as I could and especially when it made sense.

I tried so many new things in math this year; it was like a "how can I make this hands-on or more engaging" question was always being posed in the back of my mind, especially when it came to math. I could hardly teach a unit without figuring out how to make at least part of it hands-on, engaging, creative, and relevant.

Most of the activities I'm sharing here just seemed to "up the ante" on student engagement for the WHOLE class, which as you know is often a huge accomplishment in math class. Students' excitement towards new ways of learning was cumulatively the best part of my year.

Here's a snapshot of our year of hands-on math in pictures:

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

At the beginning of our place value unit, we made Place Value houses. I was able to incorporate collaboration, engineering, and problem solving. Students had specific value requirements (4 hundreds, 8 tens, 3 ones, etc) that they had to meet when building their houses.

Later, I would ask them to subtract numbers which meant they had to take away some of the blocks and figure out how to re-engineer their houses. I also set up some questions to require students to have to regroup and break their tens into ones or hundreds into tens.

I was super impressed with their designs and I think I sent them the message that our math class was going to be different!

You can grab your own set of base ten blocks here, in case your school doesn't have any (or enough) on hand.

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

During our Area and Perimeter unit, I used many of the activities from the Teacher Studio's Area and Perimeter Activity pack. In one of the activities, students used paper tiles to design a figure with a given area requirement.

Then, they colored the design on grid paper. I decided that we needed to take this activity to the next level by having students trace their irregular figure onto transparencies. See, irregular figures are complicated for students. I wanted them to go from concrete (making it themselves, knowing the area, finding the perimeter) to the representational phase of an irregular figure that did not contain squares meant to scaffold them in finding the area/perimeter. Is it possible to have figures with the same area and different perimeters? You betcha! and this activity surely proved that.

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

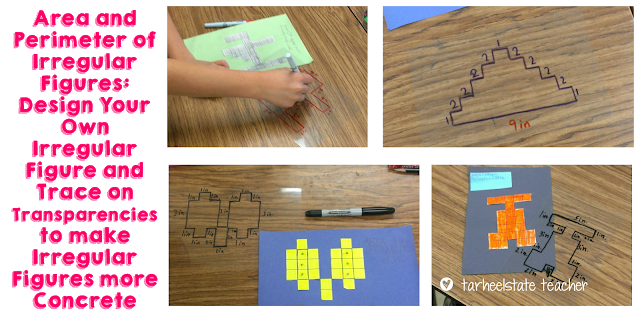
Geometry Art: it just makes sense! And my kiddos made some beautiful, cool, neato designs! After creating their design, students traced them on to paper. It was a really interesting challenge to figure out how to go from their design to a drawn copy.

I did my best to require them to make straight lines and to be as "perfectionist" as possible. Many students spent some time redoing their drawing, either at my request or because they personally want to try to make a neater copy.

I planned to have students identify different kinds of angles in their photos {you can photo copy their drawing if you want them to write on it for different purposes}, but as the activity went on, it was unnecessary. This year, I'll be thinking about how I can take this activity from a fun geometry art lesson to something that embeds more concepts. {Let me know if you have ideas for how I can do that in the comments}.

Grab your own pattern blocks from Amazon here.

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

Remember that train of thought that seemed to constantly be in the back of my mind this year? {"How can I make this hands-on or more engaging?"} Well, I s-t-r-ug-g-l-e with making measurement hands-on. I know that sounds silly because it is probably one of the most hands-on math concepts, but I don't just want my 4th graders running around measuring things. I want to go a little deeper and have a purpose.

Well, maybe I hit the mark this year. Before I started my week-long unit on length, a thought hit me: "Is fruit by the foot really a foot long?!?!?!" {I thought about using bubble tape too, but just went with Fruit by the Foot. Bubble Tape might actually be better when it comes to marking the tape so I may try that next year.}

I thought my students would be really engaged in figuring out if it was really a foot. Turns out, I don't eat enough Fruit by the Foot because the pieces are actually about 3 feet long. So, we cut our tape into two 1 foot pieces and had a third piece that was close to 1 ft leftover. We measured the third piece to see the range of lengths we had in the class. Now I'm thinking it would have been perfect to add a line plot graph to this lesson! We "blew up" an inch by marking a 6 inch piece of our Fruit Tape. We marked 1/2's, 1/4's, 1/8's, and 1/16's. It was also perfect review for equivalent fractions as we learned that marks on the ruler have different names.

Can you believe Amazon even carries Fruit by the Foot? Seriously, what don't they have?!

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

Here's the student worksheet I planned before I found out that Fruit by the Foot was NOT a foot long. The "Zooming in on an Inch" part still worked really well. You can see this student's equivalent in the picture.  

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

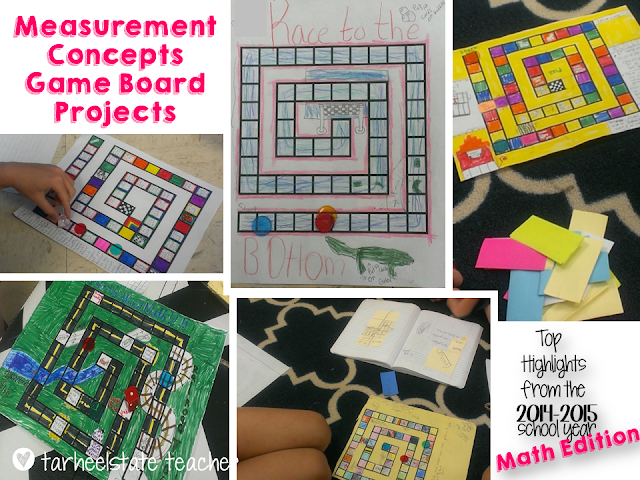
Perhaps it's harder making measurement hands on because I save that unit for right before testing. We are running out of time and I just need to hit the objectives. Close to testing and needing to spice things up? Absolutely. Game boards? Enough said! 

Well, after length we moved on to capacity. I taught the concepts (highly connecting gallons, quarts, pints, and cups with fraction concepts), we did a little practice, and then I thought, what if they practice more tomorrow by making a capacity-based game board in small groups? 

Well, this turned out to be AMAZING for two reasons: it was {surprisingly} SUPER engaging AND the kiddos created equivalence problems themselves. Can you say NO WORKSHEETS NECESSARY?!?! 

As I worked with groups of students, I focused on helping them understand how to make fractional equivalent problems like 12 cups = what fraction of a gallon? My students went above and beyond as they designed their game board and questions. They wrote up rules for their games and included answer keys. Students had 1/2 of our math time for a few days to complete their game boards, then we spent chunks of time before testing allowing them to switch games and play. {Please check out the gameboard that's named "Race to the Bottom." I just had to chuckle at that one!}

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

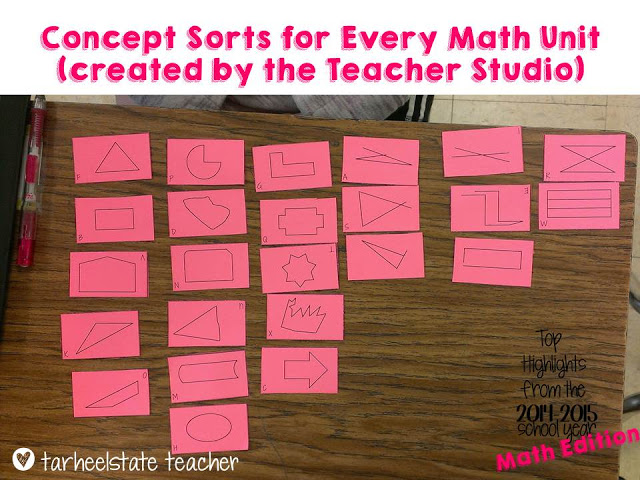
My math highlights list would not be complete without sharing the math concept sorts from Meg at the Teacher Studio that I started using this year. I'm not sure how I came across them, but I began using them to launch every unit and fell~in~love.

Concept sorts are great for a number of reasons: eliciting prior knowledge and questions, pre-assessing students' vocabulary in relation to math concepts (and the accuracy of that vocabulary knowledge), and my favorite part was when students began reviewing knowledge and teaching one another. Since they had to agree on where to place the cards as they sorted them, dialogue was critical.

I pulled out the concept sorts before, during, and after learning new concepts. Students often began with the sorts in small groups to encourage discussion. In follow up lessons, students had a copy of the sorting cards all on one sheet (not cut apart) and they recorded a sort in their math journals. (The cards are labeled with letters so it makes it really easy to discuss different ones).

With many of the sorts, I took screenshots of a lot of the cards and put them into a smartboard file so that students and I could discuss them and sort them together. Of course, I included the cards that were tricky and hotly debated when students worked in small groups. This gave me the chance to reinforce concepts and clear up any misconceptions.

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

If you missed a single highlight from my 2014-2015 Top 10 Highlights, find them all here. I've included a few freebies along the way so you definitely want to check everything out!

If you're on the lookout for great hands-on math activities for your upper elementary students, you're going to love the ideas at this blog post. Your 3rd, 4th, 5th, & 6th grade classroom or homeschool students are going to love the geometry, measurement, place value, area & perimeter, & games found here! You'll even get ideas for math concept sorts. Click through for all the details on how this can improve your math, STEAM, or project-based learning lessons. {third, fourth, fifth, sixth grader}

What did you do this year in math that felt really engaging? Did you try a new hands-on math idea that I need to include next year? Please shout it out in the comments below.

Tammy@tarheelstateteacherend of year, math9 Comments